By Nick Touran, Ph.D., 2014-04-27
You may have heard that the Earth is 4.54 billion years of age. This was ascertained by taking exact estimations of things in the soil and in shooting stars and utilizing the standards of radioactive rot to decide an age. This page will demonstrate to you how that was finished.
Basics of radiogenic isotope geography
Radioactive nuclides rot with a half-life. In the event that the half-existence of a material is 100 years and you have 1 kg of it, a long time from now you will just have 0.5 kg of it. The rest will have rotted into an alternate nuclide (called a little girl nuclide). A few radioactive nuclides exist in nature with half-lives sufficiently long to be valuable for geologic dating.
How about we experience a case of ascertaining the age of a stone with the radioactive nuclide Rubidium-87 (Rb87). This nuclide rots to Strontium-87 (Sr87) with a half-existence of 48.8 billion years. Envision going route back in time and taking a gander at some magma that is cooling to end up noticeably a stone. It normally has distinctive convergences of Rb and Sr in various parts of the stone in light of the fact that these don't really blend flawlessly. This is indicated schematically in Figure 1. When it cools the distance and takes shape, it is viewed as "conceived" and molecules can never again come in or out of the framework. Now, its radiometric clock begins ticking.
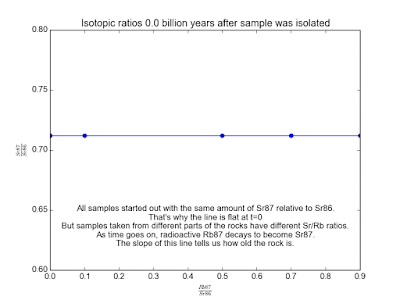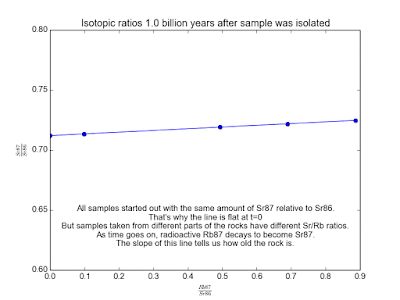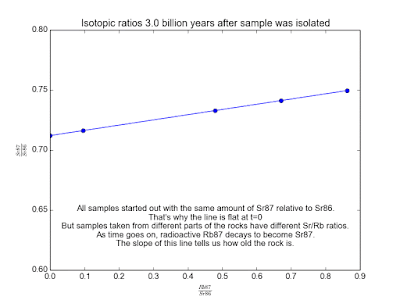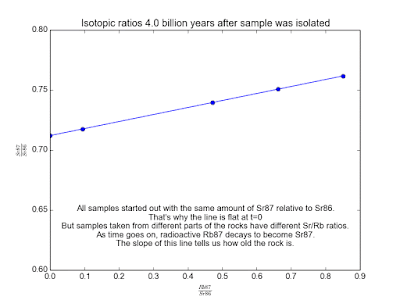
In spite of the fact that the Rb and Sr fixations contrast, it's sheltered to accept that the isotopic cosmetics of Sr and of Rb is the same all over. This is the way to making sense of how much time has gone since the stone cemented. Over the long haul, the Rb87 in the stone gradually transforms into Sr87. Parts of the stone that have more Rb87 will wind up with more Sr87. By measuring a couple of tests of the stone and looking at the relative measures of Sr87 and Rb87, we can make sense of how old the stone is!
The science of radioactive rot demonstrates to us that the quantity of Sr87 nuclides that exist after some time t is:
Sr87now=(eλt−1)Rb87now+Sr87orig
 We can measure
We can measure
We plot all our measurements and then fit a line through them. The slope of the line can then be solved for t , giving us the age of the rock. As a bonus, the intercept (b ) of the line tells us the value of Sr87orig because we know the line was flat when the age of the rock was zero. The animation in Figure 2 shows the flat line and how it increases with time.
The isochron technique can decide the age of any stone, yet new shakes are framed constantly. So to make sense of the age of the Earth, we need to look elsewhere... in the sky!
The age of the Earth
Earth has a liquid magma layer and plate tectonics, so the "shut framework" prerequisite of these radiometric dating strategies is some of the time hard to fulfill for Earth itself. Shooting stars, then again, have been skimming around in space since the close planetary system was shaped. When they come colliding with Earth, examination of their creation can be geographically broke down.
Claire Patterson was the first to precisely date the crystallization of Earth to 4.55 +/ - 0.05 billion years prior. He utilized a lead isotope isochron technique utilizing estimations from three distinct shooting stars (lead-206, lead-207 are the possible rot results of uranium-238 and uranium-235). He at that point took estimations from the profound sea that fell unequivocally on the shooting star isochron, proposing that the Earth and the shooting stars were both made in the meantime, 4.55 billion years prior. (You may have gained from COSMOS that he likewise was among the first to comprehend the general wellbeing dangers of lead sullying from leaded fuel).
Numerous different techniques have been utilized to date the Earth, with a wide range of sets of radioactive nuclides (and different strategies). They are on the whole reliable with Patterson's estimation. This is the manner by which we know how old the Earth is.
The numerical points of interest of the lead-lead isotopic clock are less direct than those of the Rb-Sr strategy. Then again, since just lead is included (rather than two concoction species), the lead-lead clock is strong against circumstances where the examples were as of late weathered or something else "opened". We will skirt the isochron induction, yet you can discover it elsewhere[1]. The final product is that the slant of the isochron with Pb207 focuses charted against Pb206 (both in respect to non-radiogenic Pb204) is equivalent to:
m=NU235NU238eλU235t−1eλU238t−1
The best-fit line appeared in Figure 3 has an incline of 0.602459. Sadly, the geochron condition above is supernatural, which means there's no mathematical answer for it and it must be settled numerically. Advantageously, this is extremely trifling with a couple of lines of Python. Utilizing this python program that I composed, I am ready to right away ascertain the age of the Earth to be 4.51 billion years utilizing lead isotopic estimations alone. Cool! This is the vulnerability guaranteed by Patterson.
Note that Uranium-235 rots to Lead-207 and that Uranium-238 rots to Lead-206. There are brief points of interest in these rot chains, however, they don't make a difference on the timescales we're managing. See the rotting steel in the See Also area underneath for rot chain points of interest.
Cell-based dating
Cell-based dating is regularly examined. It includes the radioactive nuclide Carbon-14 (otherwise known as radiocarbon) rotting to Nitrogen-14 with a 5730-year half-life. As you can envision, this half-life is short concerning the age of the Earth, so it can't be utilized to date shakes. However, it is unmatched in its capacity to date things that used to live (bones, wood, nourishment, paper, and so forth.). At the point when a living thing bites the dust, its body quits taking-up carbon. This is undifferentiated from a stone solidifying, as the body now turns into a shut arrangement of carbon, thus a radiocarbon check begins in it. Radiocarbon dating has been utilized for living things in the 100,000 year time period. Discover more about it through our See Also interfaces underneath.
Age of the Universe
Every one of the discourses on this page so far identifies with the time of rocks and of the Earth. Be that as it may, this isn't the same as the age of the universe. Researchers have discovered much confirmation for The Big Bang that made the universe 13.8 billion years prior. This made all the building squares of molecules. From that point forward, the iotas, both vast and little have been manufactured in stars in the procedures of stellar nucleosynthesis.
Suppositions made amid radiogenic isotopic geography
There are unquestionably a few presumptions made to play out these estimations. How about we experience some of them.
The half-existences of radioactive rot have stayed consistent since the development of the Earth
This supposition, otherwise called Uniformitarianism, should be legitimized before considering the figurings on this page exact. On the off chance that the half-lives change, at that point, we're up to the creek without a paddle. Luckily, there is a considerable measure of confirmation that they don't change. The least complex of which, maybe is that geologic tickers from 5 or 6 distinctive nuclide sets give reliable outcomes for similar specimens. On the off chance that half-lives were transforming, it'd be an unfathomable fortuitous event that these autonomous techniques concur so well.
Another fun truth is that 2 billion years prior, conditions in Africa were with the end goal that a characteristic atomic reactor went basically at Oklo. The plenitudes of parting items from this reactor are quantifiable today, and they are predictable with consistent half-lives. So this at least reveals to us that the half-lives were steady for as long as 2 billion years.
The isotopic plenitudes were uniform all through the close planetary system in the first place
The way that lunar, shooting star, and earthbound specimens all have reliable isotopic conveyances generally unequivocally recommends this is valid.













No comments:
Post a Comment